
We will also study rings and fields and other abstract mathematical objects, which can be thought of as groups with additional structure. The main objects of study are groups, which are abstract mathematical objects that reflect the most basic features of many other mathematical constructions. Müller-Hoissen, Folkert, Introduction to non-commutative geometry of commutative algebras and applications in physics, in Proceedings of the 2nd Mexican School on Gravitation and Mathematical Physics, Kostanz (1998) ¡¿. Math 417 is an introduction to abstract algebra. and Perez-Lorenzana, A.,, Minimal coupling and Feynman’s proof, arXiv:quant-phy/9810088 v2. , Non-commutative worlds, New Journal of Physics 6, 2–46. , Non-commutative calculus and discrete physics, in Boundaries-Scientific Aspects of ANPA 24, 73–128. , A non-commutative approach to discrete physics, in Aspects II-Proceedings of ANPA 20, 215–238. , Space and time in discrete physics, Intl. , Noncommutativity and discrete physics, Physica D 120 (1998), 125–138. , Quantum electrodynamic birdtracks, Twistor Newsletter Number 41 Pierre, Discrete Physics and the Dirac Equation, Physics Letters A, 218, pp. Pierre, Discrete Physics and the Derivation of Electromagnetism from the formalism of Quantum Mechanics, Proc. Please see our ALEKS page for more information.

Uiuc differential geometry code#
The code is non-refundable and costs 81.00. Students are required to purchase an ALEKS code to access the course online. Kauffman, Louis H., Knots and Physics, World Scientific Pub. This course uses the ALEKS assessment and learning system.
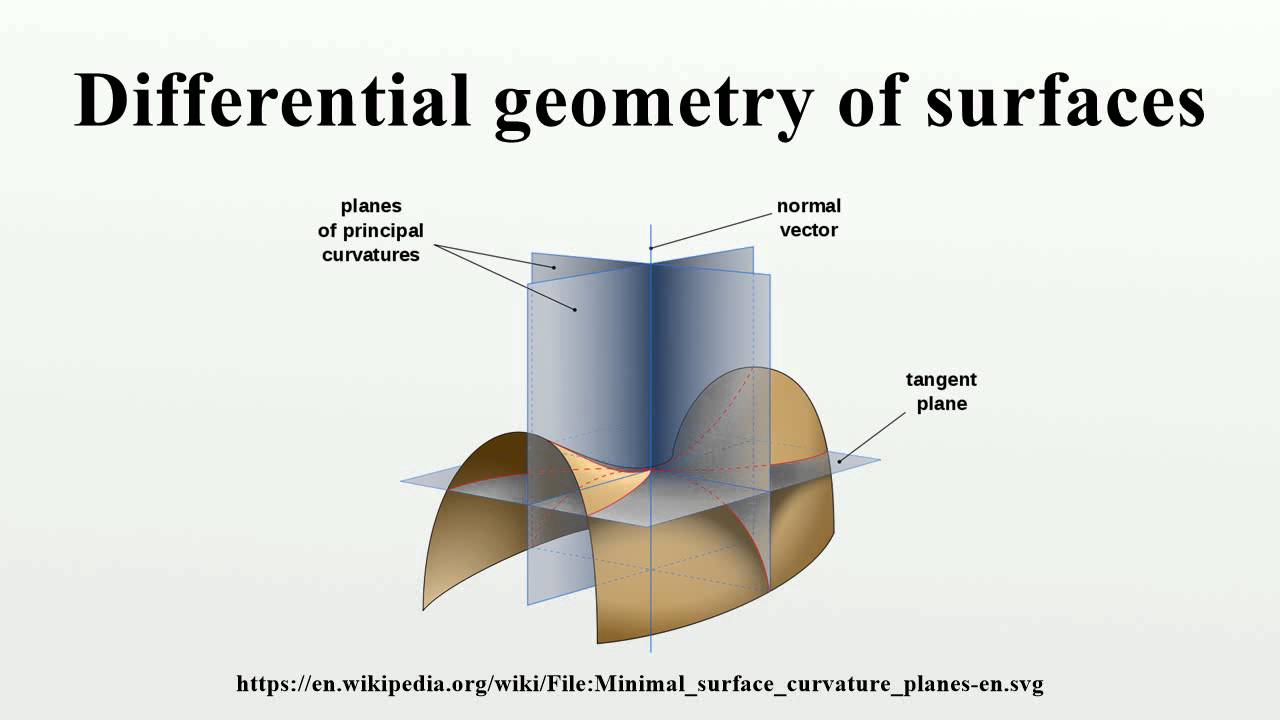
, On Feynman’s proof of the Maxwell Equations, Am. Differential geometry in computational electromagnetics, PhD Thesis, UIUC. , Quantum mechanics on a lattice and q-deformations, Phys. 58(3), March 1990, 209–211.Ĭonnes, Alain, Non-commutative Geometry Academic Press.ĭimakis, A. , Feynman’s proof of the Maxwell Equations, Am.


 0 kommentar(er)
0 kommentar(er)
